Generating Quadratic Equations
When Given Focus Vertex Directrix
If you know two of those three, you can generate a quadratic equation.
|
|
These instructions are for parabolas that have the form ax² + bx + c = 0
and are always oriented
so that they open upward "U" or downward "∩".
1) Using Focus and Directrix
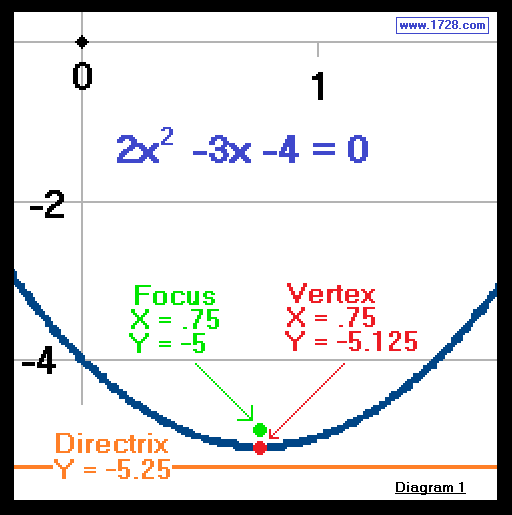
Example
We are given a focus of (.75, -5) and a directrix of Y = -5.25
What is the Quadratic Equation?
Whenever we are given two of these three:
• focus
• vertex
• directrix
We must use this formula to generate a quadratic equation:

Since we are dealing with upward-pointing or downward-pointing parabolas ("U" shaped or "∩" shaped), the "x" value of the focus will always equal the "x" value of the vertex.
Therefore the "x" value of the vertex (or "h") equals .75.
Next we have to determine the "y" value of the vertex (or "k").
(It is important to state this is NOT the value of 'p'.)
We see that the "y" value for the focus is -5 and for the diectrix it's -5.25. The vertex "y" value always lies exactly between the two and so "k" must equal -5.125.
Finally, we calculate "p" using the equations in diagram 2 and we see the distance is .125.
Now, we can enter all this information into the equation in diagram 2.
(x -h)² = 4p•(y -k)
(x -.75)² = (4•.125)•(y --5.125)
x² -1.5x + .5625 = .5•(y +5.125)
x² -1.5x + .5625 = .5y +2.5625
.5y = x² -1.5x -2
y = 2x² -3x -4
|
| |
2) Using Vertex and Directrix
Example
We are given a vertex of (.75, -5.125) and a directrix of Y = -5.25
What is the Quadratic Equation?
Knowing the vertex and the directrix is the easiest case (of the three) to generate a quadratic equation.
We don't even need to calculate the focus.

Diagram 2 tells us when the vertex is (.75, -5.125) then "h" = .75 and "k" = -5.125.
We see that "p", the vertex to directrix distance, equals .125.
We put this information into the equation and the answer is exactly the same as it is for example 1.
|
| |
|
3) Using Vertex and Focus
Example
We are given a vertex of (.75, -5.125) and a focus of (.5, -5)
What is the quadratic equation?

Looking at diagram 2, we can see from the vertex that "h"= .5 and "k" = -5.125
To get the "p" value we need the difference between the "y" value of the focus (-5) and the "y" value of the vertex (-5.125) which equals .125
Putting this information into the diagram 2 equation, we get:
(x -.75)² = (4•.125)•(y --5.125)
And from this point on, the calculations are exactly the same as in example 1.
|
"Return To Quadratic Equation Calculator"
"Return To Home Page"
Copyright © 2001 1728 Software Systems
|

