Two Angles and an Included Side Example A triangle has 2 angles of 75° and 25° and the included side is 5. What is the other angle and the other 2 sides? Let's call Angle A = 25°, Angle B = 75° and side c = 5. Angle C is easily found by: ∠C = 180° - ∠A - ∠B     = 180° - 25° - 75° ∠C = 80° Dropping a perpendicular 'hgt' (for 'height') from Angle C to side 'c', splits triangle ABC into 2 right triangles. So, angle BCD =180° - 90° - ∠B     =15° and Angle ACD =180° - 90° - ∠A     =65° Using this information and a trigonometry calculator, we can find the values of the 2 parts of line 'c'. (see diagram below). Now, the remaining calculations are quite straightforward. side a = .55535 ÷ sine(15°)   side a = .55535 ÷ .25882 side a = 2.1457 side b = 4.4447 ÷ sine(65°)   side b = 4.4447 ÷ .90631 side b = 4.9042 Two Sides and a Non-Included Angle When solving for a triangle with 2 known sides and a non-included angle, there may be no solution, one solution or even two solutions. In a triangle of sides 'c', 'a' and its opposite Angle 'A', the number of solutions is calculated by: • If a < c•sin(A) no solution • If a = c•sin(A) one solution: ∠C=90° ∠B=(90°-∠A) and side b=(c•cos(A)) • If c>a>c•sin(A) two solutions. 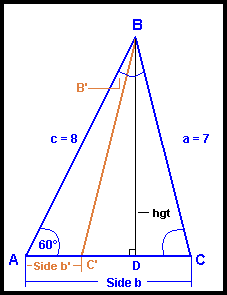• If a>=c one solution Example A triangle has sides of 7 and 8 and a non-included angle of 60° What are the other angles and the other side? Let's say side a=7, c=8 and Angle A=60° Dropping a perpendicular 'hgt' from Angle B, we find its length by: hgt = sine 60° • 8     = .86603 • 8 =   6.9282 Angle C = arc sine (hgt ÷ side a) = arc sine (6.9282 ÷ 7) = arc sine (.98974) Angle C = 81.785° Angle B is easily found by: ∠B= 180° -60° - 81.785° Angle B = 38.215° Side b = AD + DC To solve these, we'll use the Pythagorean Theorem: Side AD² = c² - hgt²   = 8² - 6.9282²   Side AD=4 Side DC² = a² - hgt²   = 7² - 6.9282²   Side DC=1 Side b = AD + DC = 4 +1   Side b = 5 Is there a second solution? If c>a>c•sin(A) there are 2 solutions. 8 > 7 > (8 • sin (60°)) Since 8 > 7 > 6.92824, there are two solutions. Whenever there are two solutions, ∠C and ∠B will each have 2 values. We will designate the "second Angle C" as C' and we find it by: ∠C' = 180° - ∠C     ∠C' = 180° - 81.789° ∠C' = 98.213° We find ∠B' by: ∠B' = 180° -∠A - ∠C'     ∠B' = 180° -60° - 98.213° ∠B' = 21.787° Since side b = AD + DC, then side b' = AD - DC = 4 - 1 Side b' = 3 For a calculator and a further explanation of side-side-angle triangles, please click here. 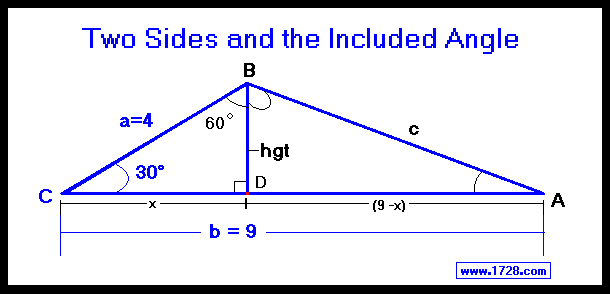Two Sides and the Included Angle Example A triangle has sides of 4 and 9 with an included angle of 30°. What are the other 2 angles and the length of the other side? Let's say side a=4 and side b=9 and angle C=30° Dropping a perpendicular 'hgt' from Angle B to side 'b', splits triangle ABC into 2 right triangles and splits side b into 'x' and '9-x'. So, angle CBD =180° - 90° - ∠C     = 60° hgt= sine 30° • 4 = .5 • 4     hgt = 2 Line segment 'x' = sine 60° • 4   'x' = .86603 • 4     'x' = 3.4641 Line DA (or 9-x) = 9 - 3.4641 = 5.5359 Arc tangent (A) = 2 ÷ 5.5359   = .36128   Arctan(A) = .36128 ∠A = 19.864° Arc tangent (DBA) = 5.5359 ÷ 2 = 2.7680   Angle (DBA) = 70.136° Angle B is found by adding CBD and ABD which equals 60° + 70.136° ∠B = 130.136° (We could have found Angle B just as easily by ∠B = 180° - ∠A - ∠C) Side c = 2 ÷ Sine 19.864° = 2 ÷ .33979   = 5.886 Side c = 5.886 (We also could have found side 'c' by using the Pythagorean Theorem). All Three Sides Of A Triangle EXAMPLE: A triangle has sides of length four, five and six. What are all 3 angles? Let's say that side a=4, side b=5 and side c=6. Yes, this could be solved in many different ways, but here's a "neat" solution. Remember Heron's formula from the triangle page? It states that triangle area = Square Root (s•(s-a)•(s-b)•(s-c)) where 's' is the semi-perimeter of the triangle. s=(4 + 5 + 6) / 2       s= 7.5 area = Square Root (7.5 •(7.5 -4)•(7.5 -5)•(7.5 -6)) area = Square Root (98.4375) area = 9.9216 So, how does this help solve the triangle? Well, there is another area formula: triangle area = ½ • base • height and therefore, height = (2 • area ÷ base) height = (2 • 9.9216 ÷ 6) height = 3.3072 ∠B = arc sine (hgt ÷ side a) ∠B = arc sine (3.3072 ÷ 4) ∠B = arc sine (.8268) ∠B = 55.771° ∠A = arc sine (hgt ÷ side b) ∠A = arc sine (3.3072 ÷ 5) ∠A = arc sine (.66144) ∠A = 41.41° We then easily solve Angle C using this equation: Angle C   =   180°   -55.771°   - 41.41° ∠C = 82.819° Go To Page One Basic Trigonometric Functions Go To Page Two Solving Oblique Triangles Using Law of Sines and Law of Cosines Return To Home Page Copyright © 2000   1728 Software Systems